step
Step response of dynamic system
Syntax
Description
step computes the step response to a step change in input
value from U to U + dU after td time
units.
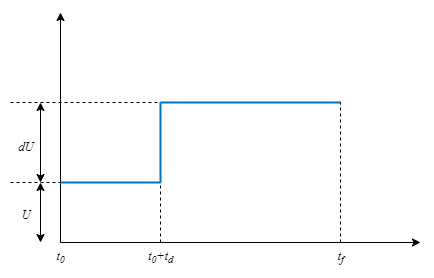
Here,
t0 is the simulation start time.
td is the step delay.
U is the baseline input value or bias.
dU is the step amplitude.
By default, the function applies step for t0 =
0, U = 0, dU = 1, and
td = 0. But, you can configure these values using
RespConfig. You can also specify the initial state
x(t0). When you don't specify
the initial state, step assumes the system is initially at rest with
input level U.
[
specifies additional options for computing the step response, such as the step amplitude
or input offset. Use y,tOut] = step(___,config)RespConfig to create config.
step(___) plots the step response of
sys with default plotting options for all of the previous input
argument combinations. For more plot customization options, use stepplot.
To plot responses for multiple dynamic systems on the same plot, you can specify
sysas a comma-separated list of models. For example,step(sys1,sys2,sys3)plots the responses for three models on the same plot.To specify a color, line style, and marker for each system in the plot, specify a
LineSpecvalue for each system. For example,step(sys1,LineSpec1,sys2,LineSpec2)plots two models and specifies their plot style. For more information on specifying aLineSpecvalue, seestepplot.
Examples
Input Arguments
Output Arguments
Tips
To simulate system responses to arbitrary input signals, use
lsim.When you need additional plot customization options, use
stepplotinstead.Plots created using
stepdo not support multiline titles or labels specified as string arrays or cell arrays of character vectors. To specify multiline titles and labels, use a single string with anewlinecharacter.step(sys,u,t) title("first line" + newline + "second line");
Algorithms
To obtain samples of continuous-time models without internal delays,
step converts such models to state-space models and discretizes them
using a zero-order hold on the inputs. step chooses the sampling time for
this discretization automatically based on the system dynamics, except when you supply the
input time vector t in the form t = T0:dt:Tf. In that
case, step uses dt as the sampling time. The resulting
simulation time steps tOut are equisampled with spacing
dt.
For systems with internal delays, Control System Toolbox™ software uses variable step solvers. As a result, the time steps
tOut are not equisampled.
References
[1] L.F. Shampine and P. Gahinet, "Delay-differential-algebraic equations in control theory," Applied Numerical Mathematics, Vol. 56, Issues 3–4, pp. 574–588.
Version History
Introduced before R2006aSee Also
Functions
impulse|RespConfig|initial(Control System Toolbox) |lsim|stepplot
Apps
- Linear System Analyzer (Control System Toolbox)