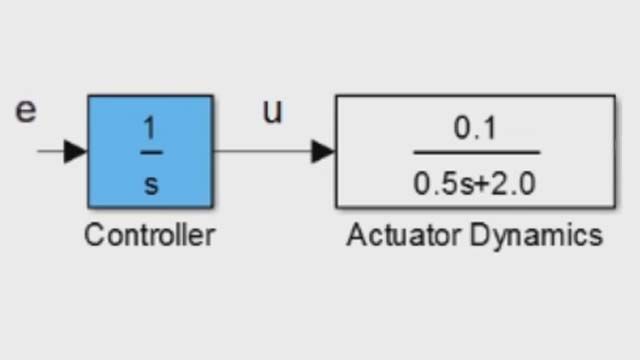
What Is a Transfer Function?
A transfer function is a convenient way to represent a linear, time-invariant system in terms of its input-output relationship. It is obtained by applying a Laplace transform to the differential equations describing system dynamics, assuming zero initial conditions. In the absence of these equations, a transfer function can also be estimated from measured input-output data.
Transfer functions are frequently used in block diagram representations of systems and are popular for performing time-domain and frequency-domain analyses and controller design. The key advantage of transfer functions is that they allow engineers to use simple algebraic equations instead of complex differential equations for analyzing and designing systems.
Examples and How To
Software Reference
See also: Bode plot, linearization, root locus, control systems, PID control, PID tuning, control design software, Time Series Regression